Peenie Wallie: 91/11: The Mathematical Terrorist

« The Kidapult | Main | 1943 Guide to Hiring Women »

February 23, 2008

91/11: The Mathematical Terrorist

It was either late 1988 or early 1989 when I started reading The Mathematical Tourist by Ivars Peterson.

On page 18, Peterson wrote

Alice knows the original number, 91, and she looks for all numbers less than 91 that generate a remainder of 30 when divided into her number. In this case, she can do it by trial and error, but mathematicians use a faster method based on the Chinese remainder theorem, which provides a handy way to reconstruct integers from certain remainders. She finds two pairs: ±11 and ±24. She can send either 11 or 24 to Bob. One of the numbers is Bob's number, but Alice doesn't know which one of the two is his.

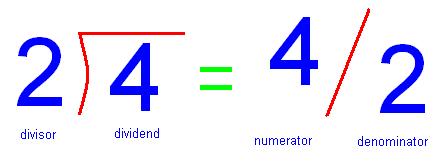
Being 19 at the time, it hadn't been that long since I had to do long division in school. I was pretty sure that 91/11 is 8 remainder 3, and 91/24 is 3 remainder 13. Since the book said otherwise, I figured I was doing something wrong. Perhaps "divided into" meant I had the dividend and divisor backwards, but reversing them didn't work either (i.e. "11/91" and "24/91"). My inability to solve this problem prevented me from understanding the rest of the chapter, and I eventually quit reading the book, since I couldn't get past page 18.

Earlier this week, I completed PGP Corporation's "Certified Technician" training course. Since the introductory material for that course dealt with public key cryptography -- a subject that Alice and Bob seem to appear in repeatedly -- I dusted off my nearly 20-year-old mostly-unread copy of The Mathematical Tourist to re-read that section.

It has been more than a few years since I've done long division by hand. So I looked up a web page about long division, and remainders, just to make sure I hadn't forgotten any important details. When was the last time any of us dealt with remainders rather than fractions?

Following the text in the book produced the same results it did about 19 years ago.

At this point, I was wondering if I was the only person stupid enough to not understand a simple passage about dividing two numbers. So I entered the first six words of the paragraph into a search engine, and found this web page with the chapter on-line.

Here is the passage as it appears on the web:

Alice knows the original number, 91, and she looks for all numbers less than 91 that generate a remainder of 30 when squared then divided into her number. In this case, she can do it by trial and error, but mathematicians use a faster method based on the Chinese remainder theorem, which provides a handy way to reconstruct integers from certain remainders. She finds two pairs: ±11 and ±24. She can send either 11 or 24 to Bob. One of the numbers is Bob's number, but Alice doesn't know which one of the two is his.

(emphasis added)

"When squared" before doing the division! Well, that's a big difference! 11²/91 = 1 remainder 30, and 24²/91 = 6 remainder 30.

I'm sure there's a lesson in all of this, about careful editing and clarity of language, and the power of a world-wide network, that I'll leave to the reader. As for me, I finally took care of one annoyance that's been nagging me in the back of my mind for almost 20 years.

Update: the fourth paragraph and the eleventh paragraph were edited to correct errors caught by an alert reader; the reversal of the numerators and denominators. I wrote them down correctly on paper when doing the long-division by hand, but reversed the divisor and dividend when typing them in fraction-format (eg, "11/91" is now "91/11" etc.) because I was reading and typing left-to-right. It's not an excuse for my carelessness, just an explanation. I'm sure there's a lesson about the irony of making typographical errors while writing about typographical errors.